In this article, you’ll learn What is Vibration? And different types of vibration and their working are all explained with pictures.
Also, you can download the PDF file at the end of this article.
What is Vibration?
Vibration is a mechanical phenomenon causing oscillations around a fixed point. The vibration occurs when elastic bodies, like springs, are displaced from their equilibrium (fixed) positions due to external forces.
This is because when a body is moved by external application, internal forces in the form of elastic or tension energy are present. These try to return the body to its original position when the pressure is released.
All of the elastic energy in the body is converted into kinetic energy when the body reaches equilibrium, causing it to move in the opposite direction. As a result, the body is again able to achieve equilibrium due to the conversion of kinetic energy into elastic or strain energy.
Thus, the vibrational motion is repeated, and energy is exchanged. Now, let’s understand the different types of vibrations with the examples below.
Read Also: Mechanical Properties That Every Engineer Should Know
Types of Vibration
Following are the types of vibrations:
- Free or natural vibration
- Forced vibration
- Damped vibration
#1 Free or Natural Vibration
Also known as natural vibration, it occurs when no external force has been applied to the body after a displacement has been given. The frequency of this type of vibration is called free or natural frequency.
These vibrations occur when a machine is started in motion with no initial input and allowed to vibrate freely. The machine vibrates at one or more natural frequencies before becoming stable. The resolution of free vibration is usually approximately sinusoidal. The amplitude appears to decrease with time.
Examples of Free Vibration:
- Swinging a child back and letting it go.
- Tapping and letting the tuning fork sound.
- The oscillation of the simple pendulum.
- Musical instrument notes.
- Fluctuations of an object attached to a horizontal spring.
Read Also: What are the different types of metals and their uses?
Types of Free Vibration
Free vibrations are further classified into three types, which are described below:
- Longitudinal vibration
- Transverse vibration
- Torsional vibration
#1 Longitudinal Vibration

When vibration has applied, the particles of the shaft or disc travel parallel to the axis of the shaft; this is known as longitudinal vibration.
In this condition, the shaft is alternately lengthened and shortened, creating tensile and compressive stresses in the shaft. For a sound wave traveling through the air, these vibrations are described as longitudinal.
#2 Transverse Vibration
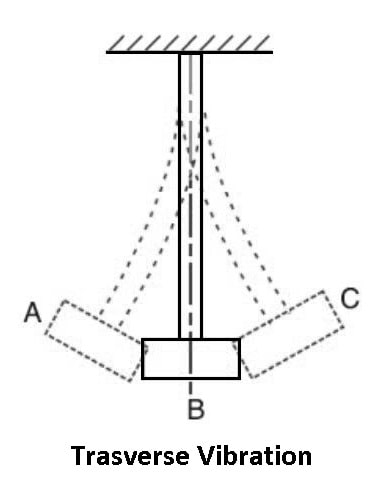
When vibration is applied, the particles of the shaft or disc move back and forth in a direction perpendicular to the shaft axis; this is known as transverse vibration. In this case, the shaft is straightened and bent, causing bending stress in the shaft.
#3 Torsional Vibration
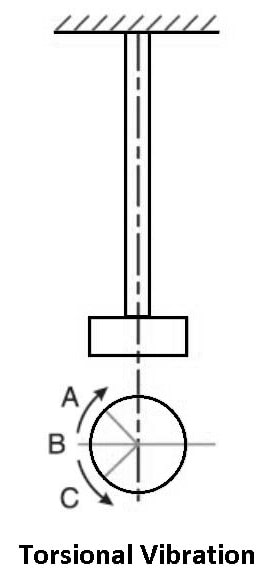
When vibration is applied, the particles of the shaft or disc rotate around the axis of the shaft; it is called torsional vibration. In this way, the shaft is twisted and separated alternately, creating torsional shear stress in the shaft.
Read Also: What is Mechanical Measurement? Definitions & Terms Used to Measurements
#2 Forced Vibration
When a mechanical system is subjected to a time-varying disturbance (load, displacement, velocity, or acceleration), forced vibration occurs. Or, when a machine vibrates under external force, the machine is said to be under forced vibration.
External forces acting on the body are periodic disturbances caused by imbalances. The frequency of these vibrations is proportional to the force applied. A resonance occurs when the external force has the same frequency as the natural vibrations. In this type of vibration, it is observed that the amplitude remains constant concerning time.
Examples of Forced Vibration:
- A harmonic or non-harmonic disturbance can be used as a periodic input for the forced vibration.
- Shaking of washing machine due to imbalance.
- Transport vibrations that are induced by an engine or uneven road.
- The vibration of a moving vehicle is forced vibration, as the engine, springs, road, etc., continue to vibrate it.
- Buildings or apartments vibration during an earthquake.
Read Also: Types of Furnaces: Their Working & Applications
#3 Damped Vibration
The damping vibration of a body is periodic and has decreasing amplitude when it is accompanied by resistance forces. Or, when there is a decrease in amplitude at each vibration, the motion is said to be damped vibration.
This is because a certain amount of energy is always dissipated near the vibrating system in order to overcome the frictional resistances of motion.
In this types of vibration, it is observed that the amplitude suddenly decreases with time. These types of vibration include music produced by a tuning fork at a greater distance. The vibrations of the Earth’s surface are damping-free in the absence of an external force.
A damping force is typically assumed to be proportional to the vibration velocity at low speeds and proportional to the square of vibration velocity at high speeds.
F∝ V at a lower speed
F∝ V2 at a higher speed
C = damping force per unit velocity (damping coefficient)
ωn = frequency of undamped vibrations

Degree of dampness:
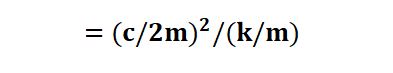
Damping factor:
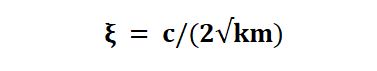
Damping Coefficient
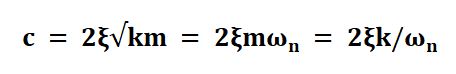
When ξ = 1, it is damping critical, thus under critical damping conditions
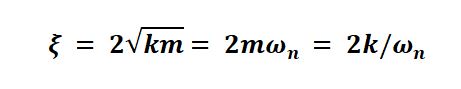
ξ = c/cc = Actual or Critical damping coefficient
- (ξ > 1) it means the system is overdamped
- (ξ < 1) it means the system is underdamped
- ωd = ωn√(1-ξ2)
Note:
- In a critically damped system, the displaced mass returns to a state of rest without oscillation in the shortest possible time.
- Undamped systems (ξ = 0) vibrate at their own natural frequency, which is determined by the constant deflection at their mass center.
- In critical damping (ξ = 1); ωd = 0 and Td = ∞. No vibrations occur in this system, and the mass ‘m’ slowly returns to equilibrium.
- An overdamped system (ξ > 1), It behaves similarly to the critical damping system.
Examples of Damped Vibration:
- Oscillation of a tree branch.
- Shock absorbers installed in the vehicle’s suspension system reduce the movement of the springs.
- The sound produced by a tuning fork over a long distance.
Read Also: What Are Different Types of Pulleys? [PDF]
Methods of Finding Natural Frequency
There are several methods that can be used to find the natural frequency, which are described below:
- Equilibrium method
- Energy method
- Rayleigh’s method
#1 Equilibrium Method
It is the principle that when a vibrating system is in equilibrium, the algebraic sum of the forces and moments acting on it is zero. This corresponds to d’Alembert’s principle, which states that the inertial forces and external forces acting on a body in equilibrium must be zero.
Let consider,
- Δ = static deflection
- k = Stiffness of the spring
Inertial force = ma
(upwards, a = acceleration)
Spring force = kx (upwards)
Now the equation we will get is,
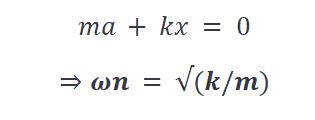
Linear frequency fn = (1/2π)√(k/m)
Time period T = 1/fn = 2π√(m/k)
#2 Energy Method
It is the principle wherein the total mechanical energy, i.e., the sum of the kinetic and potential energies, remains constant in a conservative system (without any damping).
d/dt (K.E+ P.E.) = 0
#3 Rayleigh’s Method
According to this principle, the maximum kinetic energy at a mean position would be equal to the maximum potential energy (or strain energy) at an extremum position.
The displacement of mass ‘m’ from the mean position is given by
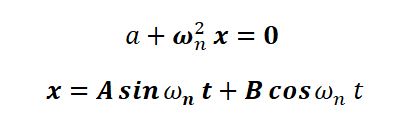
Let A = X cos φ; B = X Sin φ
Velocity,
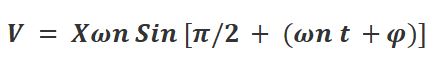
Acceleration,
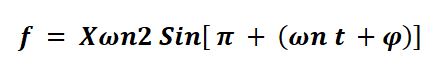
These above relations show that
- The velocity vector moves the displacement vector forward by π/2.
- The acceleration vector moves the displacement vector forward by π.
Now, consider, ‘m’ = man of the spring wire per unit length
l = length of the spring wire m1 = m’l
KE of the spring = 1/3 * KE of a mass equal to a spring moving with the same velocity as the free end.
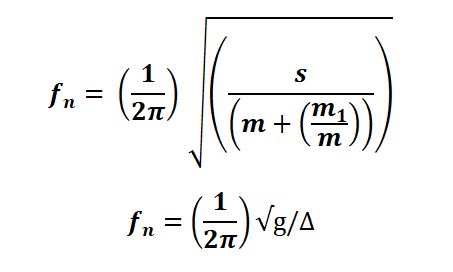
Read Also: Types of Fasteners: Their Uses & Examples [Explained]
Purpose of Vibration Analysis
The objectives of why vibration analysis is necessary are as follows:
- Vibration can cause extreme failure in machines and structures.
- Its analysis is essential to minimize vibration through the proper design of machines and their mountings.
- It is done to improve the efficiency of some machining, casting, forging, and welding processes.
- Vibration causes rapid wear as well as excessive noise.
- Due to the vibration, the metal gives a poor surface finish in the cutting process.
- This is done to study earthquake flares for geological research and in the design of nuclear reactors.
Closing It Up
That’s it. Thanks for reading. I hope I have covered everything about the “Types of Vibration.” If I missed something, or if you have any doubts, let me know in the comments. If you liked this article, please share it with your friends.
Want free PDFs direct to your inbox? Then subscribe to our newsletter.
Download PDF of this article:
You might like to explore more interesting articles in our blog: